3 Chapter 3 – Time value of Money

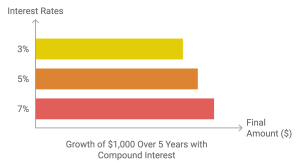
Let’s explore how an investment of $1,000 at a 5% annual interest rate grows over 5 years when compounded annually.

•Year 1:

$$FV = 1,000 \times (1 + 0.05)^1 = 1,050$$

After the first year, your investment grows by $50 in interest, resulting in $1,050.

•Year 2:

[latex]FV = 1,000 \times (1 + 0.05)^2 = 1,102.50[/latex]

In the second year, interest is earned on both the original $1,000 and the $50 from Year 1, adding another $52.50, bringing the total to $1,102.50.

•Year 3:

[latex]FV = 1,000 \times (1 + 0.05)^3 = 1,157.63[/latex]

By the third year, the interest earned grows to $55.13, further boosting the total to $1,157.63.

•Year 4:

[latex]FV = 1,000 \times (1 + 0.05)^4 = 1,215.51[/latex]

The interest earned in Year 4 is $57.88, increasing the total to $1,215.51.

•Year 5:

[latex]FV = 1,000 \times (1 + 0.05)^5 = 1,276.28[/latex]

Finally, in Year 5, the interest earned is $60.77, bringing the total future value to $1,276.28.

Key insights from This Example:

1. The Power of Compounding: Interest is earned not only on the principal but also on accumulated interest, resulting in exponential growth over time.
2. Patience Pays Off: The longer your money stays invested, the more pronounced the effect of compounding becomes.
3. Visualizing Growth: Over 5 years, the total interest earned adds up to $276.28, or 27.6% of the original $1,000 investment.

Interactive Question for Students:

•How much would your investment grow to in 10 years instead of 5 years at the same rate? Use the formula:

[latex]FV = 1,000 \times (1 + 0.05)^{10}[/latex]

Suppose you want to have $1,000 in 5 years, and the annual interest rate is 5%, compounded annually. How much should you invest today to achieve that goal?

Step-by-Step Calculation

To calculate Present Value (PV), use the formula:

$$ PV = \frac{FV}{(1 + r)^n} $$

Where:

•FV = 1,000 (Future Value you want in 5 years)

•r = 0.05 (Annual interest rate as a decimal)

•n = 5 (Number of years)

1. Substitute the Values:

$$ PV = \frac{1,000}{(1 + 0.05)^5} $$

2. Calculate the Denominator:

$$(1 + 0.05)^5 = 1.27628$$

3. Divide to Find PV:

$$ PV = \frac{1,000}{1.27628} \approx 783.53 $$

Result

You need to invest $783.53 today to have $1,000 in 5 years at an annual interest rate of 5%.

What Happens with a Different Interest Rate?

If the interest rate is 10% instead of 5%, the PV changes:

1. Recalculate Denominator:

$$(1 + 0.10)^5 = 1.61051 $$

2. Divide to Find PV:

[latex]PV = \frac{1,000}{1.61051} \approx 620.92[/latex]

With a 10% interest rate, you only need to invest $620.92 today to reach $1,000 in 5 years.

1. Saving for a Goal:

You’re planning a purchase that will cost $1,000 in 3 years, and you can earn 4% annual interest on your savings. How much should you save today?

• Use FV = 1,000, r = 0.04, n = 3:

$$PV = \frac{1,000}{(1 + 0.04)^3} = \frac{1,000}{1.12486} \approx 889.00$$

You need to save $889.00 today.

2. Paying Off Debt:

You owe $1,000 in 2 years, and the lender offers to accept an early payment at a 6% discount rate. How much do you need to pay today?

• Use FV = 1,000, r = 0.06, n = 2:

$$PV = \frac{1,000}{(1 + 0.06)^2} = \frac{1,000}{1.1236} \approx 890.00$$

You’d need to pay $890.00 today.

Interactive Question for Students

•If you want $1,000 in 10 years, and the annual interest rate is 8%, how much should you invest today?

•Use the formula:

$$PV = \frac{FV}{(1 + r)^n}$$

Scenario

You invest $5,000 in a savings account that offers an 8% annual interest rate, compounded monthly. You plan to leave the money untouched for 3 years. What will the Future Value (FV) of your investment be after 3 years?

Step-by-Step Calculation

The formula for Future Value (FV) with non-annual compounding is:

[latex]FV = PV \times \left(1 + \frac{r}{m}\right)^{n \times m}[/latex]

Where:

•PV = 5,000 (Present Value or initial investment)

•r = 0.08 (Annual interest rate as a decimal)

•m = 12 (Number of compounding periods per year, monthly compounding)

•n = 3 (Number of years)

1. Calculate the Monthly Interest Rate (r/m):

[latex]\text{Monthly Interest Rate} = \frac{0.08}{12} = 0.0066667[/latex]

2. Determine the Total Number of Compounding Periods (n \times m):

[latex]\text{Total Periods} = 3 \times 12 = 36[/latex]

3. Substitute the Values into the FV Formula:

[latex]FV = 5,000 \times \left(1 + 0.0066667\right)^{36}[/latex]

[latex]FV = 5,000 \times (1.0066667)^{36}[/latex]

4. Raise the Base to the Power of 36:

Using a calculator or software:

[latex](1.0066667)^{36} \approx 1.28368[/latex]

5. Multiply by the Present Value (PV):

[latex]FV = 5,000 \times 1.28368 \approx 6,418.40[/latex]

Result

After 3 years, your investment will grow to approximately $6,418.40.

Scenario:

An account offers an 8% annual nominal interest rate, compounded monthly. What is the EAR?

1. Identify variables:

[latex]r = 0.08, \, m = 12[/latex]

2. Substitute into the formula:

[latex]EAR = \left(1 + \frac{0.08}{12}\right)^{12} - 1[/latex]

3. Calculate monthly interest rate:

[latex]\frac{0.08}{12} = 0.0066667[/latex]

4. Solve for EAR:

[latex]EAR = \left(1.0066667\right)^{12} - 1 \approx 0.083 = 8.3\%[/latex]

Result:

An 8% nominal interest rate, compounded monthly, is equivalent to an 8.3% EAR.

Background:

Mark, a recent college graduate, has started a new job and wants to invest his savings in an account that offers the best return. He has narrowed down his options to two banks, each offering different interest rates and compounding frequencies:

• • Bank A offers an annual interest rate of 8%, compounded quarterly.
  • Bank B offers an annual interest rate of 7.9%, compounded monthly.

Mark needs to determine which bank account will give him the highest effective return over the course of a year.

Objective:

Students will use the formulas for Future Value with Non-Annual Compounding and Effective Annual Rate (EAR) to analyze Mark’s options and decide which bank account is more beneficial.

Task:

• Calculate the Effective Annual Rate (EAR) for each bank account:
  • Bank A: 8% compounded quarterly.
  • Bank B: 7.9% compounded monthly.
• Determine which bank account provides the highest effective annual rate and explain why it would be the better investment for Mark.

Formulas:

[latex]EAR = \left(1 + \frac{r}{m}\right)^m - 1[/latex]

Calculation:

Bank A (8% compounded quarterly):

r = 0.08

m = 4

[latex]EAR = \left(1 + \frac{0.08}{4}\right)^4 - 1 \approx 8.24\%[/latex]

Bank B (7.9% compounded monthly):

r = 0.079

m = 12

[latex]EAR = \left(1 + \frac{0.079}{12}\right)^{12} - 1 \approx 8.17\%[/latex]

Questions for Discussion:

• Based on the EAR calculations, which bank should Mark choose?
• Why is the EAR important when comparing investment or loan offers?
• How would the decision change if the compounding frequency at Bank B were increased to daily?

Reference:

To explore the importance of compounding frequency and interest rates, students can refer to Investopedia’s article on Effective Annual Rate.

1. Retirement Planning:

Starting early with small, consistent investments can lead to substantial savings over time, thanks to the power of compound interest.

•Example:

If you contribute $200 per month to a retirement account earning 6% annual interest (compounded monthly) for 30 years, how much will you save?

$$FV = PMT \times \frac{\left(1 + \frac{r}{m}\right)^{n \times m} – 1}{\frac{r}{m}}$$

Where:

• PMT = 200
• r = 0.06, m = 12, n = 30

$$FV = 200 \times \frac{\left(1 + \frac{0.06}{12}\right)^{12 \times 30} – 1}{\frac{0.06}{12}}$$

$$FV = 200 \times \frac{(1.005)^360 – 1}{0.005} \approx 200 \times \frac{6.022575 – 1}{0.005}$$

$$FV \approx 200 \times 1,004.52 \approx 200,904$$

You will accumulate approximately $200,904 over 30 years, illustrating the importance of starting early.

2. Loan Comparisons:

Understanding TVM helps in comparing loan options to choose the one with the lowest effective cost.

•Example:

A fixed-rate mortgage with a lower monthly payment might appear attractive, but factoring in total interest paid over the loan’s life using PV calculations provides a clearer picture.

1. Investment Decisions:

Businesses rely on TVM to assess whether projects or investments will add value to the firm.

•Example:

A company evaluates purchasing new machinery costing $100,000, which will generate $30,000 annually for 5 years. If the discount rate is 8%, the Present Value (PV) of cash inflows can be calculated to determine if the investment is worthwhile.

$$PV = \sum \frac{CF_t}{(1 + r)^t}$$

Where:

• CF_t = 30,000, r = 0.08, t = 1 to 5
• $$PV = \frac{30,000}{1.08^1} + \frac{30,000}{1.08^2} + \frac{30,000}{1.08^3} + \frac{30,000}{1.08^4} + \frac{30,000}{1.08^5}$$
• $$PV \approx 27,778 + 25,720 + 23,815 + 22,059 + 20,425 \approx 119,797$$

Since the PV of cash inflows (119,797) exceeds the cost (100,000), the investment adds value to the company.

2. Capital Budgeting:

Small business owners often use TVM to decide between immediate and deferred investments.

•Example:

Should a company buy new software for $20,000 today or wait two years for an improved version costing $22,000? Assuming a 5% discount rate, calculate the PV of the future cost:

$$PV = \frac{FV}{(1 + r)^n}$$

$$PV = \frac{22,000}{(1 + 0.05)^2} \approx \frac{22,000}{1.1025} \approx 19,951$$

Since the PV of the future cost is slightly less than $20,000, it may be worth waiting, especially if the improved software provides added value.