As discussed earlier, hydronium and hydroxide ions are present both in pure water and in all aqueous solutions, and their concentrations are inversely proportional as determined by the ion product of water (K_w). The concentrations of these ions in a solution are often critical determinants of the solution’s properties and the chemical behaviors of its other solutes, and specific vocabulary has been developed to describe these concentrations in relative terms. A solution is neutral if it contains equal concentrations of hydronium and hydroxide ions; acidic if it contains a greater concentration of hydronium ions than hydroxide ions; and basic if it contains a lesser concentration of hydronium ions than hydroxide ions.

A common means of expressing quantities, the values of which may span many orders of magnitude, is to use a logarithmic scale. One such scale that is very popular for chemical concentrations and equilibrium constants is based on the p-function, defined as shown where “X” is the quantity of interest and “log” is the base-10 logarithm:

pX = -log X

The pH of a solution is therefore defined as shown here, where [H₃O⁺] is the molar concentration of hydronium ion in the solution:

pH = -log [H₃O⁺]

Rearranging this equation to isolate the hydronium ion molarity yields the equivalent expression:

[H₃O⁺] = 10^-pH

Likewise, the hydroxide ion molarity may be expressed as a p-function, or pOH:

[H₃O⁺] = -log [OH^–]

or

[OH^–] = 10^-pOH

Finally, the relation between these two ion concentration expressed as p-functions is easily derived from the K_w expression:

K_w = [H₃O⁺] [OH^–]
-log K_w = -log([H₃O⁺] [OH^–]) = -log [H₃O⁺] + -log [OH^–]
pK_w = pH +pOH

At 25 °C, the value of K_w is 1.0 × 10⁻¹⁴, and so:

14.00 = pH +pOH

As was shown in the previous section, the hydronium ion molarity in pure water (or any neutral solution) is 1.0 × 10⁻⁷ M at 25 °C. The pH and pOH of a neutral solution at this temperature are therefore:

pH = -log [H₃O⁺] =  -log (1.0 × 10⁻⁷) = 7.00

pOH = -log [OH^–] =  -log (1.0 × 10⁻⁷) = 7.00

And so, at this temperature, acidic solutions are those with hydronium ion molarities greater than 1.0 × 10⁻⁷ M and hydroxide ion molarities less than 1.0 × 10⁻⁷ M (corresponding to pH values less than 7.00 and pOH values greater than 7.00). Basic solutions are those with hydronium ion molarities less than 1.0 × 10⁻⁷ M and hydroxide ion molarities greater than 1.0 × 10⁻⁷ M (corresponding to pH values greater than 7.00 and pOH values less than 7.00).
Since the autoionization constant K_w is temperature dependent, these correlations between pH values and the acidic/neutral/basic adjectives will be different at temperatures other than 25 °C. For example, the “Check Your Learning” exercise in the previous section showed the hydronium molarity of pure water at 80 °C is 4.9 × 10⁻⁷ M, which corresponds to pH and pOH values of:

pH = -log [H₃O⁺] =  -log (4.9 × 10⁻⁷) = 6.31

pOH = -log [OH^–] =  -log (4.9 × 10⁻⁷) = 6.31

At this temperature, then, neutral solutions exhibit pH = pOH = 6.31, acidic solutions exhibit pH less than 6.31 and pOH greater than 6.31, whereas basic solutions exhibit pH greater than 6.31 and pOH less than 6.31. This distinction can be important when studying certain processes that occur at nonstandard temperatures, such as enzyme reactions in warm-blooded organisms. Unless otherwise noted, references to pH values are presumed to be those at standard temperature (25 °C) (Table 1).

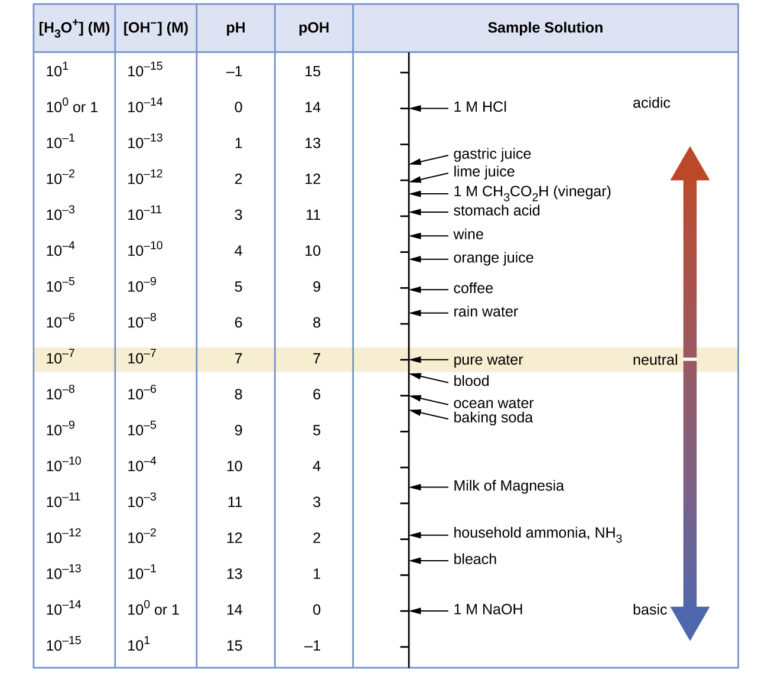
Figure 1 shows the relationships between [H₃O⁺], [OH⁻], pH, and pOH, and gives values for these properties at standard temperatures for some common substances.

Note: ENVIRONMENTAL SCIENCE

Normal rainwater has a pH between 5 and 6 due to the presence of dissolved CO₂ which forms carbonic acid:

H₂O(l) + CO₂(g) → H₂CO₃(aq)

H₂CO₃(aq) ⇌ H⁺(aq) + HCO₃^–(aq)

Acid rain is rainwater that has a pH of less than 5, due to a variety of nonmetal oxides, including CO₂, SO₂, SO₃, NO, and NO₂ being dissolved in the water and reacting with it to form not only carbonic acid, but sulfuric acid and nitric acid. The formation and subsequent ionization of sulfuric acid are shown here:

H₂O(l) + CO₂(g) → H₂CO₃(aq)

H₂SO₄(aq)  → CO₂(g) H₂CO₃(aq)

Carbon dioxide is naturally present in the atmosphere because we and most other organisms produce it as a waste product of metabolism. Carbon dioxide is also formed when fires release carbon stored in vegetation or when we burn wood or fossil fuels. Sulfur trioxide in the atmosphere is naturally produced by volcanic activity, but it also stems from burning fossil fuels, which have traces of sulfur, and from the process of “roasting” ores of metal sulfides in metal-refining processes. Oxides of nitrogen are formed in internal combustion engines where the high temperatures make it possible for the nitrogen and oxygen in air to chemically combine.

Acid rain is a particular problem in industrial areas where the products of combustion and smelting are released into the air without being stripped of sulfur and nitrogen oxides. In North America and Europe until the 1980s, it was responsible for the destruction of forests and freshwater lakes, when the acidity of the rain actually killed trees, damaged soil, and made lakes uninhabitable for all but the most acid-tolerant species. Acid rain also corrodes statuary and building facades that are made of marble and limestone (Figure 2). Regulations limiting the amount of sulfur and nitrogen oxides that can be released into the atmosphere by industry and automobiles have reduced the severity of acid damage to both natural and manmade environments in North America and Europe. It is now a growing problem in industrial areas of China and India.

For further information on acid rain, visit this website hosted by the US Environmental Protection Agency.

 

The acidity of a solution is typically assessed experimentally by measurement of its pH. The pOH of a solution is not usually measured, as it is easily calculated from an experimentally determined pH value. The pH of a solution can be directly measured using a pH meter (Figure 3).

The pH of a solution may also be visually estimated using colored indicators (Figure 4).