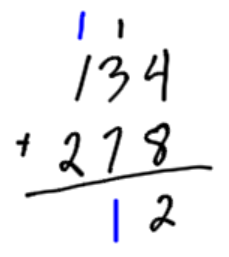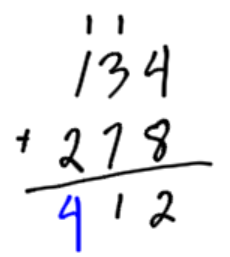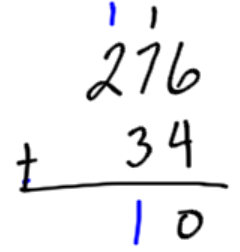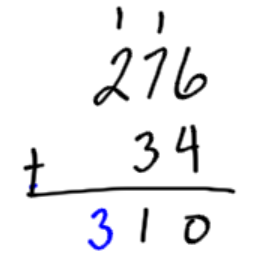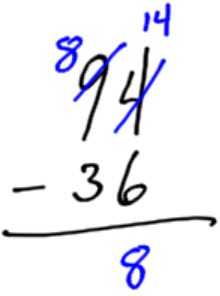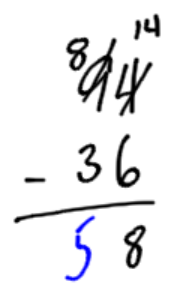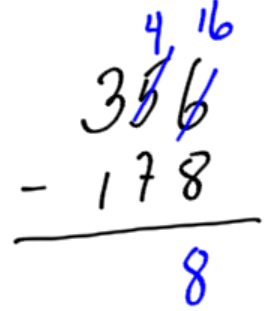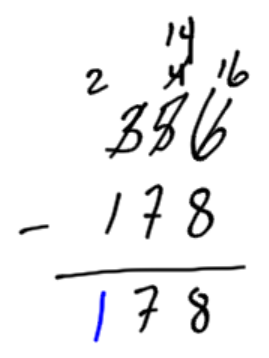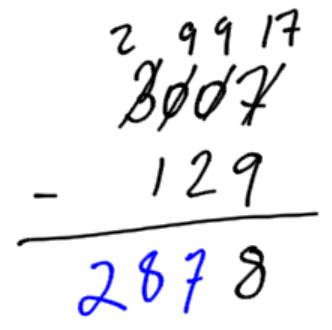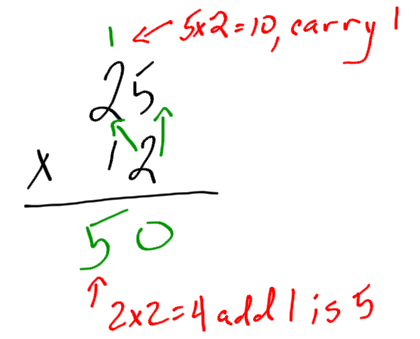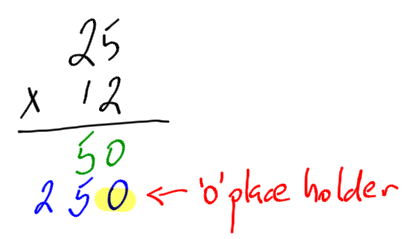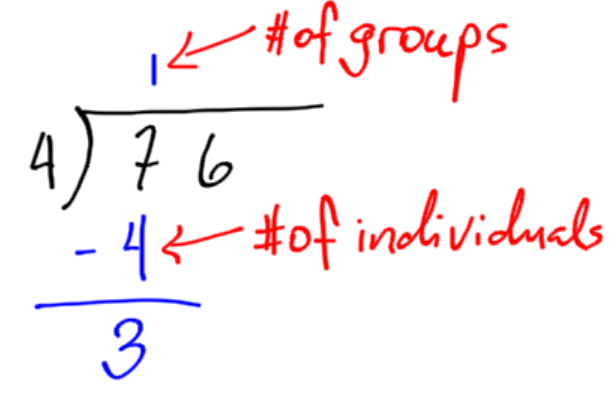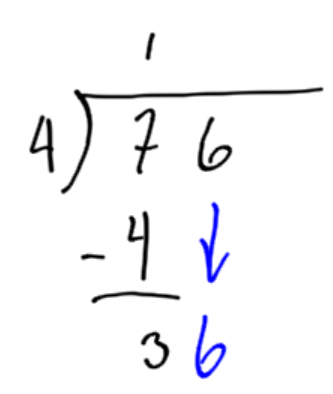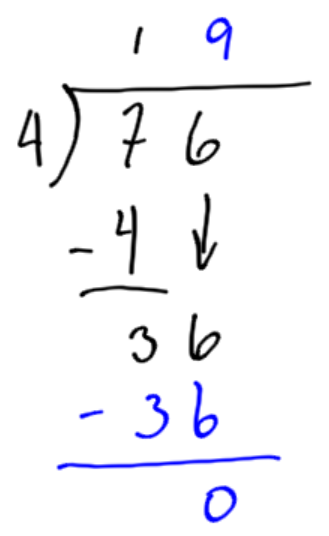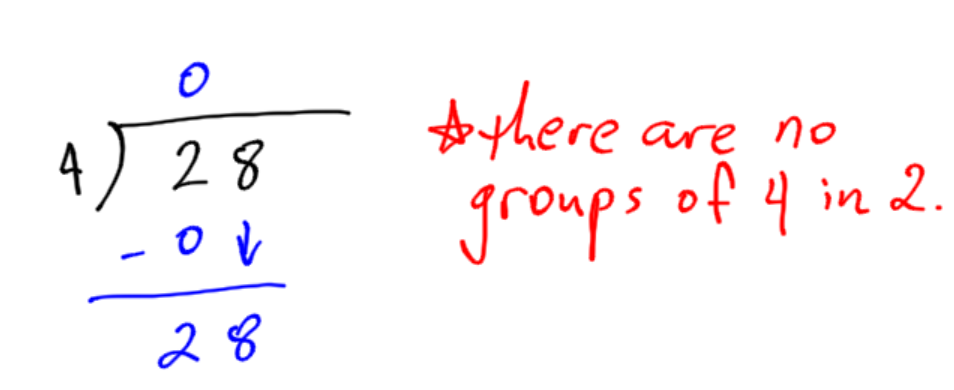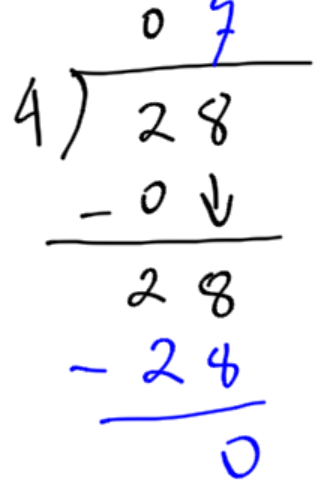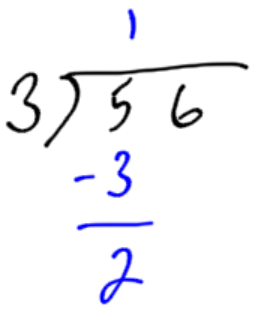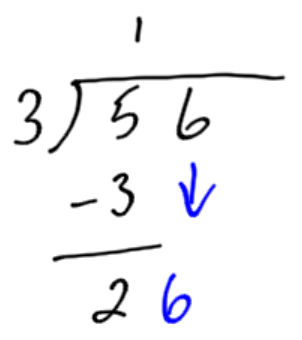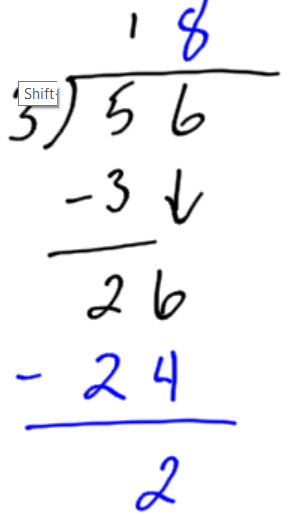1 Basic Operations

Introduction:

In this module you will learn about whole numbers, decimals, rounding, addition, carrying, subtraction, borrowing, long multiplication, long division and order of operations. You will be provided with examples of each concept, and then be given opportunities to practice.

Chapter Contents:

• Topic 1: Whole Numbers
  • 1.1 Place Value
• Topic 2: Decimal Numbers
  • 2.1 Decimals
  • 2.2 What do Decimals Really Mean?
  • 2.3 Whole Numbers and Decimals
  • 2.4 Rounding
• Topic 3: Mathematical Operations on Whole Numbers
  • 3.1 Addition and Carrying
  • 3.2 Subtraction and Borrowing
  • 3.3 Long Multiplication and Carrying
  • 3.4 Long Division
• Topic 4: Order of Operations
• Test: Outcome 1

Topic 1: Whole Numbers

---

Whole numbers are non-negative numbers without fractions or decimals.  An example of whole numbers are 0, 1, 2, 3 and so on.  Negative 30 (-30) degrees celcius is not a whole number.  7.2, or 3/4 are also not whole numbers.

1.1 Place Value

Knowing place value is important when rounding numbers. It will also help us understand why “carrying” and “borrowing” works in addition and subtraction.

If a person has 1 loony, 3 ten-dollar bills, and 2 hundred-dollar bills, we can say that person has $231 (two hundred thirty-five) dollars.

Each digit in a number has a value. The position in the number determines the value. For example, the number “231” is 1 “one” plus 3 “tens” plus 2 “hundreds”.

Here is a chart giving the names of place values:

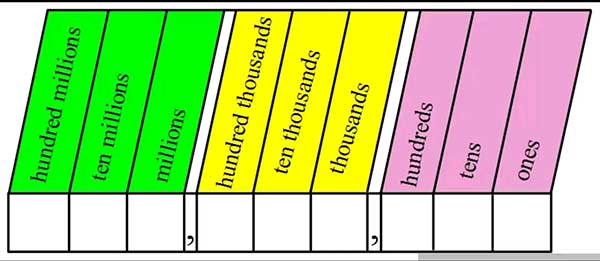This Photo by Unknown Author is licensed under CC BY-NC-ND

 

Example 1

The number 628,134,593 has:

6	Hundred-millions
2	Ten-millions
8	Millions
1	Hundred-thousands
3	Ten-thousands
4	Thousands
5	Hundreds
9	Tens
3	Ones

1.1 Practice Exercises: Whole Number Place Values

[h5p id=”43″]

Topic 2: Decimal Numbers 

---

2.1 Decimals

It is important to understand what decimals really are because you will come across them every day. Decimals represent a portion (or fraction) of a whole number.

Just like whole numbers have a place value for each digit, decimals also have a place value for each digit. The “decimals” are to the right of the period (decimal point).

This Photo by Unknown Author is licensed under CC BY

NOTE:

• Each decimal place value name ends in -th.
• There are no “oneths”. Decimals start at tenths.

Example 2

The number 0.234 is often read out as “zero point two-three-four.” This is ok; everyone will understand what you are saying. Technically, the number should be read out “two hundred thirty-four thousandths.” By reading it this way, you are saying the last digit in the number is in the thousandth position.

2.1 Practice Exercises: Decimals

[h5p id=”44″]

 

The Digit Zero Zeros written to the right of the decimal part do not change the value of the number. For example, 95.23 is the same as “95.230”. Zeros written to the left of the whole number part do not change the value of the number. For example, 95.23 is the same as “095.23”. The decimal point is not written after a whole number only for convenience. It still exists. For example, 95 is the same as “95.” or even “95.0”

2.2 What do Decimals Really Mean?

Decimals are fractions and fractions are decimals. We know generally that “one half” is the same as 0.5. Why is this?

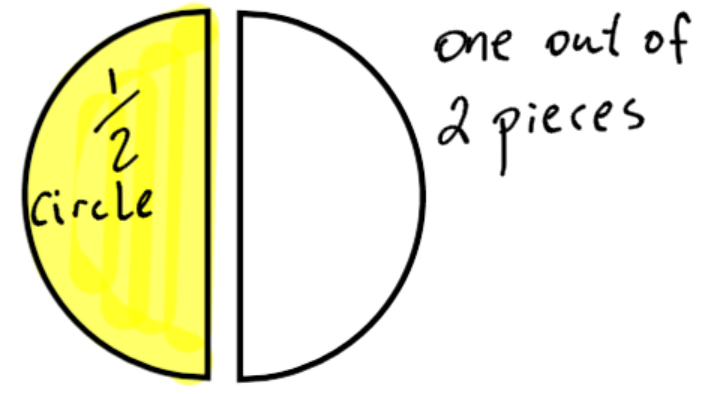
Two pieces represent the whole circle. One piece is 1 out of 2 pieces, also written as ½.

If you divide 10 into two equal pieces, each piece contains 5 numbers. Another way of saying this is “five-tenths.”

As we learned before, “five-tenths” can be written as “0.5”.

Notice the word divide from above. This is a keyword. Check-in your calculator: press 5 ÷ 10. You should see the answer: “0.5”.

In general, the way to change a fraction into a decimal is to use division. (One way to remember this is that the division sign on your calculator ÷ looks like a fraction.)

2.2 Practice Exercises: Converting Fraction to Decimal

1. Convert the following into decimals using your calculator:
   1. [h5p id=”45″]
   2. [h5p id=”46″]
   3. [h5p id=”47″]
   4. [h5p id=”48″]

 

2.3 Whole Numbers and Decimals

In the last practice question, you may have noticed that the answer had a “1” to the left of the decimal. Notice also that the top number of the fraction is larger than the bottom number. (7 is larger than 4.) This is called an “improper fraction.”

When the top number of a fraction (numerator) is larger than the bottom number (denominator), the result is more than one. Literally, you have enough pieces to make one whole, with more pieces left over.

Seven quarters is the same as one full piece of pizza with 3 pieces left over (the pieces are quarters.)

We will cover this in greater detail under the topic “Fractions.”

2.4 Rounding

Rounding is an important skill. Whenever you do a calculation with numbers, you have the potential to create a whole bunch of decimals on your calculator. Not only are all these numbers a pain to write down, but they are also meaningless.

A Grain of Sand If, for example, you are calculating the volume of sand going into some concrete, you will need to multiply three numbers together. Sand weighs about 1.6 grams per cubic centimeter. If you calculate the volume of sand to be 2352.000045 centimeters cubed, the weight of that sand will be 3763.200072 grams. If the volume of the sand were to be only 2352 centimeters cubed, the weight of the sand would be 3763.2 grams. The point is, the extra 0.000072 grams of sand is so small, it can’t even be measured by a normal scale. It is less than one grain of sand! So, using all the decimals in 2352.000045 is meaningless.

A number can be rounded off to include any number of decimal places you want.

There are three basic steps to rounding:

• Identify the “round-to” digit
• Look one digit to the right
• Decide to round up to the next “round-to” digit or keep the same “round-to” digit.

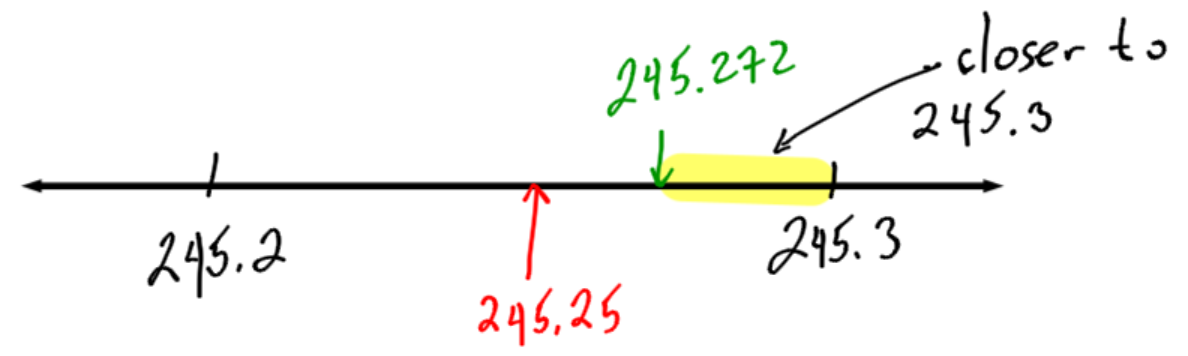
Example 3

Round 245.272 to the nearest tenth.

Step	What I am thinking
Identify the “round to” digit	We are rounding to the nearest tenth. The digit in the tenth’s spot is 2. The final answer will only have one decimal (the tenth) and it will be either 2 or 3 (depending on if we round up or down.)
Look one digit to the right	The digit to the right is 7.
Decide to round down or up	Since the digit to the right is larger than 5, it is more than half-way to 245.3. It is closer to 245.3 than 245.2. We can round up to 245.3

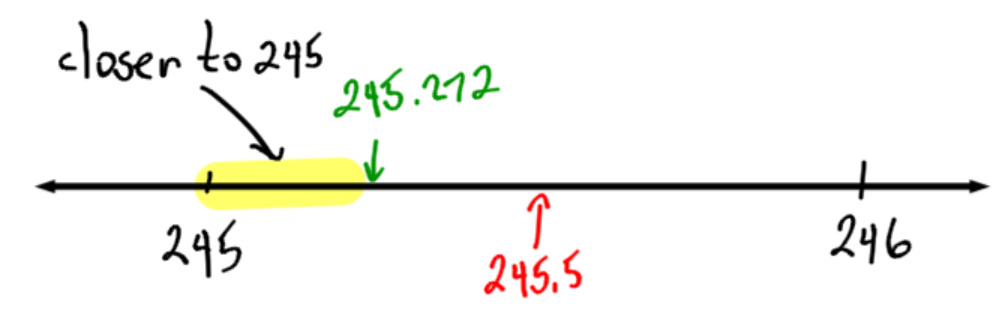
Example 4

Round 245.272 to the nearest whole number (ones).

Step	What I am thinking
Identify the “round to” digit	We are rounding to the nearest whole number (ones.) The digit in the ones spot is 5. The final answer will only have whole numbers and it will be either 5 or 6 (depending on if we round up or down.)
Look one digit to the right	The digit to the right is 2. (It doesn’t matter that it is on the other side of the decimal.)
Decide to round down or up	Since the digit to the right is less than 5, it is less than half-way to 246. It is closer to 245 than 246. We can round down to 245

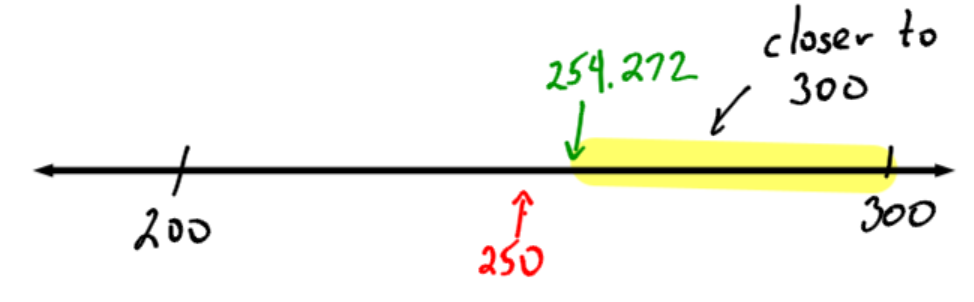
Example 5

Round 254.272 to the nearest hundred.

Step	What I am thinking
Identify the “round to” digit	We are rounding to the nearest hundred. The digit in the hundreds spot is 2. The final answer will only have whole numbers and it will be either 200 or 300 (depending on if we round up or down.)
Look one digit to the right	The digit to the right is 5. (All the rest of the digits to the right of 5 don’t play any part in this.)
Decide to round down or up	Since the digit to the right is 5, the answer could go down to 200 or to up to 300. In this case, I can look one more digit to the right: 254. The number is slightly more than halfway, so I will round up to 300.

2.4 Practice Exercises: Rounding

Round the following numbers to the given place value:

[h5p id=”49″]

Topic 3: Mathematical Operations on Whole Numbers 

---

The words “Mathematical Operations” mean addition, subtraction, multiplication, and division. In this section you will learn when to use each “operation”, and how to perform the calculations by hand.

In this section, it will be very important to remember that each digit in a number has a different value. For example, the number 523 has 3- ones, 2-tens, and 5-hundreds.

3.1 Addition and Carrying

Addition is the mathematical operation that combines amounts of the same thing. We will see in the fractions section that when adding, you can only add items of the same type. For example, if you have five apples and three oranges, you may have eight fruits, but you certainly do not have eight apples, nor eight oranges.

The word “sum” is sometimes used to mean “add”. For example: “find the sum of 3 and 5” means the same thing as “add 3 and five.”

Carrying

Maybe the easiest way to understand what is happening with carrying is to think about money. In this situation we have only loonies, ten-dollar bills, and hundred-dollar bills.

Let’s add 35 plus 96. We have five loonies plus six loonies which is in total eleven loonies. To not carry so much change, we cash in eleven loonies for one ten-dollar bill, and we have one loony left over.

Because we only can add things of the same type, we add the one ten-dollar bill in with the other ten-dollar bills. We start with three ten-dollar bills plus nine ten-dollar bills plus the one ten-dollar bill we carried over and have in total thirteen ten-dollar bills.

Example 1

Add the numbers 134 and 278. In this example, we will carry over to the tens spot and to the hundreds spot.

Step	What I am thinking
	Add the loonies. There are 4 and 8, or 12 loonies. We can cash in for a 10 dollar bill. Add the ten, to the other tens in the tens spot.
	Add all the tens together. The 3 and 7, plus the one you cashed in from before. There are a total of 11 tens. Change 10 tens in for a hundred dollar bill.
	Add all the hundreds together. The answer is 412.

 

Example 2

Add the numbers 34 and 276. In this example, we usually write the larger number on top, but it is not necessary. Also remember that the number 34 can also be written as 034.

Step	What I am thinking
	Add the 6 loonies and 4 loonies. Change that in for 1 ten dollar bill.
	Add the tens together, including the one you carried over from last step.
	Add the hundreds together including the one from last step. The answer is 310.

3.2 Subtraction and Borrowing

Subtraction is the mathematical operation that finds the space between 2 numbers.

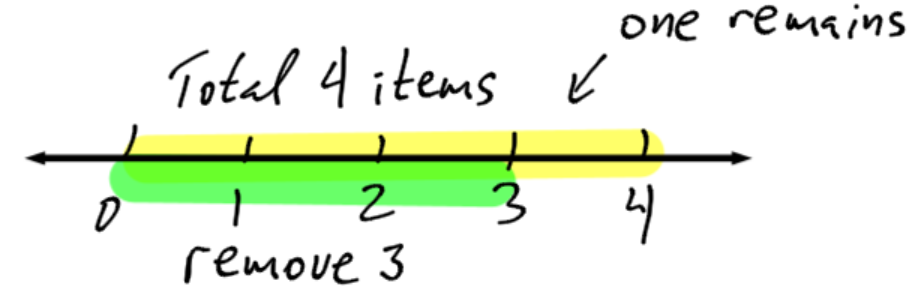
Another way to look at the same thing is to use the number line to count. Start at 4, and count to the left 3 (subtract, or negative, means move left on the number line.)

For example, you use subtraction if you give someone money and want to figure out how much you have left. You would also use subtraction if you have used up a certain amount of supplies and you want to see how much you have left.

The word “difference” is sometimes used to mean subtraction, or minus. For example, “find the difference between 32 and 26” means “32 minus 26”. Note the order you subtract numbers in makes a difference. “32 – 26” means something different than “26 – 32.”

Borrowing

Let’s continue thinking about money. We have only loonies, ten-dollar bills, and hundred-dollar bills. when we don’t have enough money to subtract from, we have to take money from another higher place value.

In the previous addition example, we needed to change all our “ones” to “tens.” When borrowing, we need to change “tens” back to “ones.” For example, subtract 36 from 94. (That is written 94 – 36.) If you have 4 loonies, there is not enough to take 6 loonies away. We “borrow” a ten-dollar bill. We need to cash this ten-dollar bill into loonies to be able to give the proper change. I now have 14 loonies (4 originally, and 10 from the 10-dollar bill I borrowed.) 14 loonies subtract 6 loonies is 8 loonies.

Step	What I am thinking
	4 is not large enough to take away 6 from. Borrow 1 ten dollar bill from the 9 (leaving 8). Cash the ten dollar bill in, and add to the four you already have, to make 14. 14 minus 6 is 8.
	Now in the tens spot, there are 8 tens, minus 3 tens leaves 5 tens. The answer is 58.

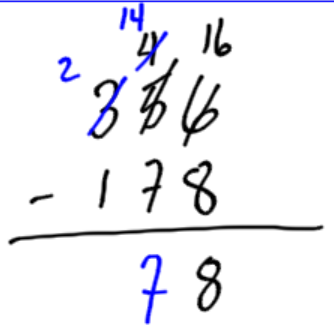
Example 3

Subtract 178 from 356 (written as 356 – 178.) In this example, we must borrow from the tens, and then again from the hundreds.

Step	What I am thinking
	Six is too small to take eight away. We must take 1 ten from the tens spot. Cash the ten dollar bill into loonies and add it to the 6 loonies you already have. 16 – 8 = 8
	Now look at the tens spot. There are 4 tens, but we are taking away 7. We must borrow a hundred, and cash in for 10 tens. Add the 10 tens to the 4 we already have. 14-7=7
	Finally, we have two hundred dollar bills, and we are taking away one hundred dollar bill. The final answer is 178.

 

Example 4

Calculate 3007 minus 129. In this example, we need to look further than just one place value to the left to borrow from

Step	What I am thinking
	The 7 is too small to take away 9. We must borrow, but there is nothing to borrow from the tens, nor the hundreds. Start by borrowing a thousand dollar bill. This sets off a chain reaction. Cash the thousand in for 10 hundreds. Borrow a hundred and cash in for 10 tens. Borrow a ten and cash in for 10 ones. Now we can take 9 away from 17.
	Now we have enough on top to do all the other subtractions. I will do them in one step. 9 tens minus two tens are 7 tens. 9 hundred minus 1 hundred is 8 hundreds. 2 thousands minus zero thousands are 2 thousands. The answer is 2878.

3.3 Long Multiplication and Carrying

Multiplication has to do with groups of things. For example, if you have five boxes, and each box contains six things, in total you have thirty things. You could come up with this answer by adding six- five times: 6 + 6 + 6 + 6 + 6 = 30. An easier way to do this is to multiply: 6 X 5 = 30. Basically, multiplication is a shortcut for adding.

You can note that the order of multiplying numbers does not matter: 6 X 5 = 30 and 5 X 6 = 30.

 

The Process of Long Multiplication

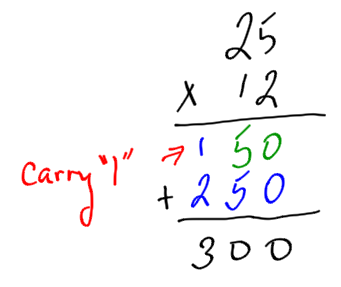
There are steps for doing long multiplication (for example 25 X 12.) In this series of steps, you need to multiply every combination of numbers. Also, the steps may involve carrying, just like in addition.

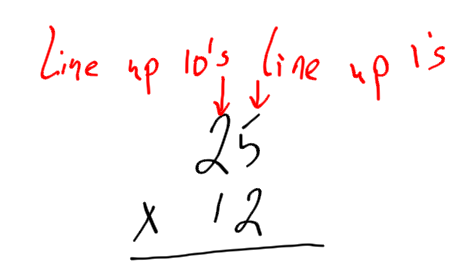
Let’s walk through this for 25 X 12.

Step	What I am thinking
1. Write the numbers one on top of the other. Put the larger number on top. Line up the ones on top of the ones, tens on top of the tens…
2. Multiply the bottom number by all the top numbers. Carry if necessary. If there is a carried number, add it in after multiplying.
3. Move to the tens. Multiply the bottom number (tens spot) by all the top numbers. Put a “0” place holder because you are multiplying by 10, not 1.
4. Add all the numbers together, using carrying if necessary.

 

Example 5

Calculate 236 X 129. In this example, we need to multiply ones, tens, and hundreds. There will be three lines to add. Remember the second line has a “0” placeholder. The third line will have a “00” place holder. Here is the work:

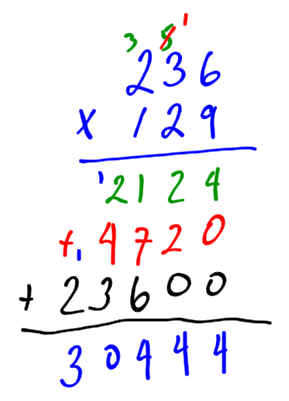
Example 6

An inch can be divided into 8 parts. Each part is called “one eighth”. How many “eighths” are there in 47 inches?

We could add 8 + 8 + 8 + … (47 more times) but this is tedious. Instead, we can use multiplication.

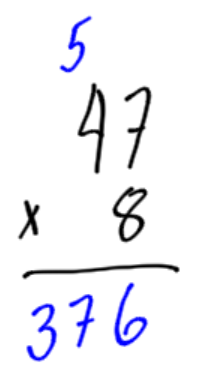
Different Cultures Think Differently The way we have done multiplication is the way we were taught in school. Remember, this is just a procedure that is followed, handed down to use from the European culture. Different cultures have different ways of understanding multiplication. The result is the same: we are adding up groups of numbers. In Asian cultures long multiplication is done completely differently because Asian cultures do not have numbers like Europeans.

Example 7

Using the line method, calculate 53 X 12

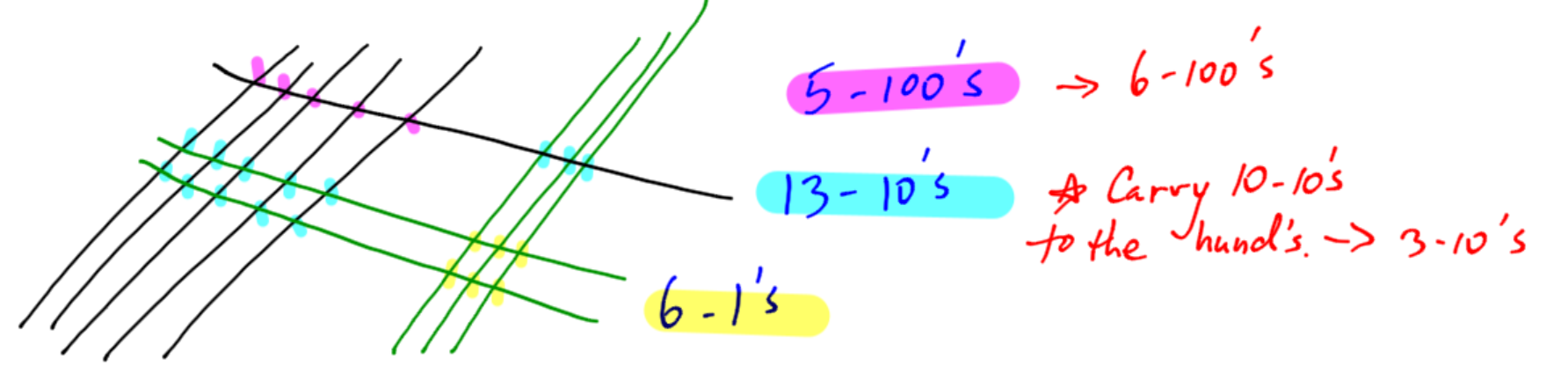
This method requires counting the intersections. The green lines are ones. The black lines are tens.

• Green cross green represents 1 x 1. There are 6 of these intersections.
• Green cross black represents 1 x 10. There are 13 of these intersections.
• The black cross black represents 10 x 10. There are 5 of these intersections.

This means there are 5- ones, 13- tens, and 6- hundreds. We still need to carry 10- tens: change them into 1-hundred and add to the 5 other hundreds.

Then answer is 636.

3.4 Long Division

Long division, in a way, is the opposite of multiplication. Remember, in multiplication, we are figuring out how many individual items there are in a bunch of groups. In division, we are finding out how many groups there are if we collect up items into bunches.

It is important to note that the order of the numbers when dividing matter (unlike multiplication.) There are also many ways of writing division. The words “12 divided by 4” can be typed into your calculator as “12 ÷ 4” or stated as “4 divided into 12” and written like “4 ⟌12”. Also, a division can be represented as a fraction:

You would use division if you wanted to break items up into groups and see how many groups there are.

Just like multiplication, division follows a series of steps that are repeated. Some of these steps may involve borrowing, just like subtraction.

Let’s walk through this for 76 divided by 4.

Step	What I am thinking
	When writing 76 divided by 4 for long division, the 4 goes on the outside. Ask myself: How many groups of 4 are there in 7? There is 1 group of 4, with 3 left over. Write the 1 on top of the 7. Subtract to find the difference of 3.
	Bring the next digit down and put it beside the leftover from the previous step.
	Now we need to find out how many groups of 4 are there in 36. Well, 4 x 9 is 36, so there are nine complete groups of four in 36. There are no more numbers to divide, so the answer is 19.

 

Example 8

Calculate 28 divided by 4. In this example, we can see what happens when the first number is too small to divide into.

Step	What I am thinking
	Two is not big enough to have any groups of 4. Put a 0 down because there are no groups of 4. Bring down the 8 as before. *Note: you can skip over this step when you realize the first digit is too small.
	There are 7 groups of 4 in 28 (4 x 7 = 28). Write the 7 above the eight. Subtract the answer to find the left over difference. In this case, there is no left over difference.

 

Example 9

Calculate 56 divided by 3. In this example we can see what happens when a number does not exactly divide evenly into another. We get a remainder.

Step	What I am thinking
	There is one group of 3 in 5, with 2 left over.
	Bring down the 6 to create the next number to divide: 26
	8 groups of 3 items are 24 items. We have 26 items, so there will be two leftover items not in a group. The answer is 18, remainder 2.

Practice Exercises 3.1 – 3.4: Mathematical Operations on Whole Numbers and Decimals

1. What mathematical operation (add, subtract, multiply, divide) would you use in these situations:
   1. You have 90 m between poles and 56 poles. How many meters of the line do you need?
   2. There are 180 degrees total in a triangle. Two of the angles combined are 112 degrees. How many degrees is the third angle?
   3. There is a spool of 1000 m of cable. The space between poles is 90 m. How many poles will one spool of cable cover?
2. Do the following by hand. Check your answer with your calculator.
   1. 1982 + 2399
   2. 3402 – 1929
   3. 2345 X 188
   4. 167 ÷ 12
3. Additional Practice

1 6783 4793 + 6329

2 7403 + 4932

3 67208 + 56256

4 49973 2670 + 367

5 75037 + 56200

• Subtraction:

6 670943 – 569340

7 50376 – 36455

8 67034 – 43578

9 734 – 67

10 3490 – 1678

• Multiplication:

674 × 340

7893 × 38

2001 × 78

734 × 167

28 × 46

• Division: give the answer with the remainder.

180 ÷ 90

894 ÷ 6

6739 ÷ 45

256 ÷ 8

400 ÷ 145

3.1 – 3.4 Practice Exercises Solutions: 

1a. 4381, b. 68, c. 11. 2a. 4381, b. 1473, c. 440860, d. 13 remainder 11

-Answers for addition, subtraction, and multiplication not given- check with a calculator.

5 Division: 16. 2, 17. 149, 18. 149 remainder 34, 19. 32, 20. 2 remainders 22 remainders 110

Topic 4: Order of Operations

---

In some mathematical calculations, you will need to do several operations in a row to come up with the answer. There is a proper order to do these in. This is called the “order of operations.” (Remember the word “operations” means add, subtract, multiply, or divide.) You may have heard of the term “P. E. D. M. A. S.” or “B. E. D. M. A. S.” This is a way to remember what order to do the operations in. Another way to remember the order of operations is the phrase “Please Excuse My Dear Aunt Sally.”

When doing these questions, do them in multiple steps. In each step, complete one set of operations. Write each step on a new line. Then, compare your answer with an answer obtained with your calculator.

Example 1

Calculate (6 + 7) x 2 using the order of operations.

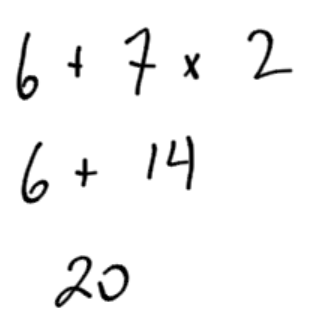
Example 2

Calculate (6 + 7) x 2 using the order of operations.

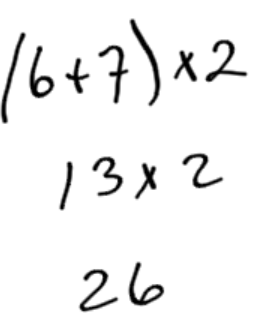
Example 3

Calculate 11 – 3 x 3 + 4 x 2 using the order of operations.

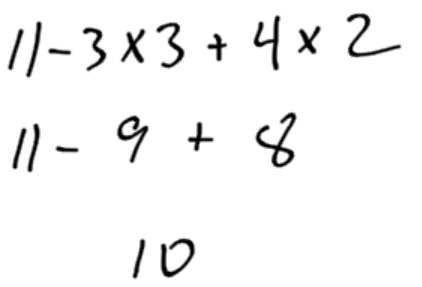
Example 4

Calculate 3 + 2² x 5 using the order of operations.

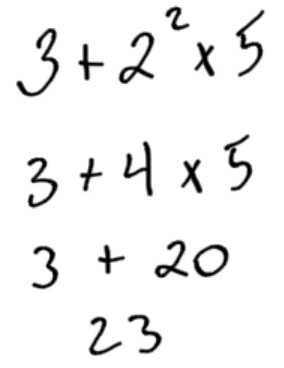
Example 5

Calculate (4 + 1)² + 3 x 2 using the order of operations.

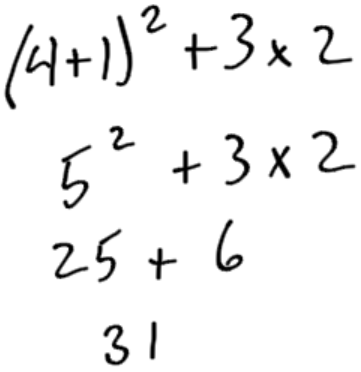
Practice Exercises 4.1: Order of Operations

[h5p id=”66″]

4.1 Practice Exercises Solutions (step-by-step):

1. 
2. 
3. 
4. 
5. 
6. 
7. 
8. 
9. 
10. 

Outcome 1 Test

Complete the Essentials 1 Math: Basic Operations Chapter Quiz on Brightspace.