6 Tolerance, Precision, and Accuracy
Section Information
Outcome/Competency: You will be able to determine tolerance, precision, and accuracy using the appropriate measuring tools.
Timing: 6h
Rationale: Why is it important for you to learn this skill?
In the real world, there is no such thing as “5 feet.” Something might be close enough to 5 feet to call it that, but if you use a more accurate ruler, you may find it is 5 feet and 1/16 inches. Depending on the application, that 1/16 inch might make all the difference in the world. In manufacturing and the trades, it is essential to realize that it is difficult, if not impossible, to have something measure an exact value. All we can do is indicate an acceptable range of values and use instruments that are accurate enough to measure that range of values. Tolerance, precision, and accuracy is the language you will need to use to describe this situation.
Objectives:
To be competent in this area, the individual must be able to:
- Describe tolerance, precision, and accuracy and the relationship between all three
- Round numbers to the given place value
- Determine the accuracy of a multimeter and represent calculations with an equivalent accuracy
Learning Goals
- Identify and describe precision, accuracy, tolerance and their relationship
- Solve problems using rounding numbers to given place values using the correctly identified scale of measurement
Introduction:
In this section you’ll learn about accuracy and precision of a measurement, uncertainty, precision of measuring tools, and tolerance. You’ll be presented with concepts and examples and then given opportunities to practice your skills.
Chapter Contents:
Topic 1: Tolerance, Precision and Accuracy
An old rusted double-pan balance is shown with a weighing stone on one pan:

Figure 1. When the bar that connects the two pans is horizontal, then the masses in both pans are equal. The “known masses” are typically metal cylinders of standard mass such as 1 gram, 10 grams, and 100 grams. (credit: Serge Melki).
A digital analytical balance:

Figure 2. Many mechanical balances, such as double-pan balances, have been replaced by digital scales, which can typically measure the mass of an object more precisely. Whereas a mechanical balance may only read the mass of an object to the nearest tenth
1.1 Accuracy and Precision of a Measurement
Science is based on observation and experiment—that is, on measurements. Accuracy is how close a measurement is to the correct value for that measurement. For example, let us say that you are measuring the length of a standard computer paper. The packaging in which you purchased the paper states that it is 11.0 inches long. You measure the length of the paper three times and obtain the following measurements: 11.1 in., 11.2 in., and 10.9 in. These measurements are quite accurate because they are very close to the correct value of 11.0 inches. In contrast, if you had obtained a measurement of 12 inches, your measurement would not be very accurate.
The precision of a measurement system refers to how close the agreement is between repeated measurements (which are repeated under the same conditions). Consider the example of the paper measurements. The precision of the measurements refers to the spread of the measured values. One way to analyze the precision of the measurements would be to determine the range, or difference, between the lowest and the highest measured values. In that case, the lowest value was 10.9 in. and the highest value was 11.2 in. Thus, the measured values deviated from each other by at most 0.3 in. These measurements were relatively precise because they did not vary too much in value. However, if the measured values had been 10.9, 11.1, and 11.9, then the measurements would not be very precise because there would be significant variation from one measurement to another.
The measurements in the paper example are both accurate and precise, but in some cases, measurements are accurate but not precise, or they are precise but not accurate.
Example 1
A GPS system that is attempting to locate the position of a restaurant in a city. Think of the restaurant location as existing at the center of a bull’s-eye target and think of each GPS attempt to locate the restaurant as a black dot. In Figure 3 you can see that the GPS measurements are spread out far apart from each other, but they are all relatively close to the actual location of the restaurant at the center of the target. This indicates a low-precision, high-accuracy measuring system. However, in Figure 4 the GPS measurements are concentrated quite closely to one another, but they are far away from the target location. This indicates a high precision, low accuracy measuring system.
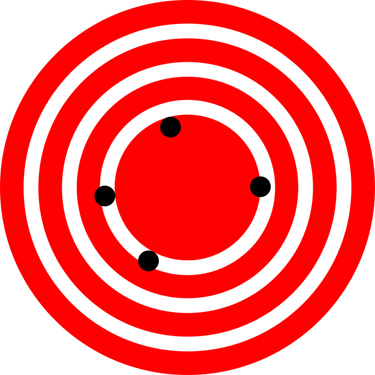
Figure 3. A GPS system attempts to locate a restaurant at the center of the bull’s-eye. The black dots represent each attempt to pinpoint the location of the restaurant. The dots are spread out quite far apart from one another, indicating low precision.

Figure 4. In this figure, the dots are concentrated rather closely to one another, indicating high precision, but they are rather far away from the actual location of the restaurant, indicating low accuracy. (credit: Dark Evil).
1.1 Practice Exercises
1. A millimeter ruler is used to measure a gaff on climbing gear. The gaff was measured by five people, and each person read 21 mm, 20 mm, 20 mm, 21 mm, and 20 mm. Is this measurement precise? Accurate?
2. An inch ruler is used to measure the same. Each person measured the gaff at 1 inch.
a) Is this measurement precise? Why?
b) Is this measurement accurate? Why?
Solution:
1. These measurements are accurate because of the small scale used to measure. The increments of a millimeter can closely match the true length of the gaff. The measurement may not be so precise because there was a variation between 21 mm and 20 mm
2.a) This measurement is precise because repeated measurement yields all the same result.
b) It is not accurate because an inch is a large increment and can not accurately represent the true length of the gaff.
1.2 Accuracy, Precision and Uncertainty
The degree of accuracy and precision of a measuring system are related to the uncertainty in the measurements. Uncertainty is a quantitative measure of how much your measured values deviate from a standard or expected value. If your measurements are not very accurate or precise, then the uncertainty of your values will be very high. In more general terms, uncertainty can be thought of as a disclaimer for your measured values.
Example 2
If someone asked you to provide the mileage on your car, you might say that it is 45,000 miles, plus or minus 500 miles. The plus or minus amount is the uncertainty in your value. That is, you are indicating that the actual mileage of your car might be as low as 44,500 miles or as high as 45,500 miles, or anywhere in between. All measurements contain some amount of uncertainty. In our example of measuring the length of the paper, we might say that the length of the paper is 11 in., plus or minus 0.2 in. In our paper example, the length of the paper could be expressed as 11 in. ± 0.2 in.
The factors contributing to uncertainty in a measurement include:
- Limitations of the measuring device,
- Irregularities in the object being measured,
- Any other factors that affect the outcome (highly dependent on the situation).
In our example, such factors contributing to the uncertainty could be the following: the smallest division on the ruler is 0.1 in., or one side of the paper is slightly longer than the other. At any rate, the uncertainty in measurement must be based on careful consideration of all the factors that might contribute and their possible effects.
1.3 Precision of Measuring Tools
Uncertainty is a critical piece of information, both in physics and in many other real-world applications. Imagine you are caring for a sick child. You suspect the child has a fever, so you check his or her temperature with a thermometer. What if the uncertainty of the thermometer were 3.0 °C? If the child’s temperature reading was 37.0 °C (which is normal body temperature), the “true” temperature could be anywhere from a hypothermic 34.0 °C to a dangerously high 40.0 °C. A body thermometer with an uncertainty of 3.0 °C would be useless. On the other hand, a thermometer attached to the window on a house, used to measure the outdoor temperature, would be acceptably accurate with an uncertainty of 3 degrees.
An important factor in the accuracy and precision of measurements involves the precision of the measuring tool. In general, a precise measuring tool is one that can measure values in very small increments. For example, a standard ruler can measure length to the nearest millimeter, while a caliper can measure length to the nearest 0.001 millimeter. The caliper is a more precise measuring tool because it can measure extremely small differences in length. The more precise the measuring tool, the more precise and accurate the measurements can be.

Figure 5. This photo by Unknown Author is license under CC.
In this wooden ruler, the smallest measurement that can be made is one millimeter. Sources of poor precision might be the width of the markings.
In the case of a caliper (shown below), a much more precise measurement can be taken. A digital read out eliminates the loss of precision caused by thick measurement lines. The instrument itself is constructed to measure to a much smaller increment.

When we express measured values, we can only list as many digits as we initially measured with our measuring tool. For example, if you use a standard ruler to measure the length of a stick, you may measure it to be 36.7 cm. You could not express this value as 36.71 cm because your measuring tool was not precise enough to measure a hundredth of a centimeter.
1.3 Practice Exercises
[h5p id=”54″]
1.4 Tolerance
When manufacturing, there is always a small degree of variation in size. The nominal value is the perfect size of the object. Because, in reality, there is always a small variation in size. It is necessary to list what an acceptable range is. For example, when producing nuts and bolts, if the nut is too large in diameter or the bolt is too small in diameter, they will not stay threaded together. If the nut is too small and the bolt is too thick, they will not fit either. There is an acceptable, small, range of measurements that are allowable when making nuts and bolts.
Example 3
1.4 Practice Exercises
1. What are the minimum and maximum measurements allowable?
a) 23.345 mm ± 0.005 mm
b) ![]()
c) 1500 km ± 50 km
Solution:
a) 23.340 mm to 23.350 mm
b) ![]()
c) 1450 km to 1550 km
Summary
- Accuracy of a measured value refers to how close a measurement is to the correct value. The uncertainty in a measurement is an estimate of the amount by which the measurement result may differ from this value.
- Precision of measured values refers to how close the agreement is between repeated measurements.
- The precision of a measuring tool is related to the size of its measurement increments. The smaller the measurement increment, the more precise the tool.
- Uncertainty is a quantitative measure of how much your measured values deviate from a standard or expected value. It can be expressed as a ± value after the measurement.
Outcome 6 Test
Complete the Essentials 1 Math: Tolerance, Precision and Accuracy Chapter Quiz on Brightspace.
Attribution
This information can be found for free at
http://cnx.org/contents/031da8d3-b525-429c-80cf-6c8ed997733a@9.103.
A huge thank you to Open Stax for their work.
This information was extracted and modified from https://pressbooks.bccampus.ca/practicalphysicsphys1104/chapter/1-3-accuracy-precision-and-significant-figures/
On September 12, 2021 and is used under a Creative Commons Attribution 4.0 International License.
