4
The Most Common Fundamental Units
| Dimension | Unit |
|---|---|
| Length | Meter ( m ) |
| Mass | Kilogram ( kg ) |
| Time | Seconds ( s ) |
| Temperature | Kelvin ( K ) |
Standard Prefixes
| Multiple | Prefix |
|---|---|
|
|
Tera, T |
|
|
Giga, G |
|
|
Mega, M |
|
|
Kilo, K |
|
|
Hecto, k |
|
|
Deka, da |
|
|
Deci, d |
|
|
Cent, c |
|
|
Milli, m |
|
|
Micro, |
|
|
Nano, n |
|
|
Pico, p |
Example: 1,000,000 Pa = 1 ![]() MPa
MPa
Newton’s Second Law
![]()
![]()
Sometimes, the questions indicate weight (W)
![]()
![]()
![]()
![]()
Temperature Conversion
![]()
![]()
![]()
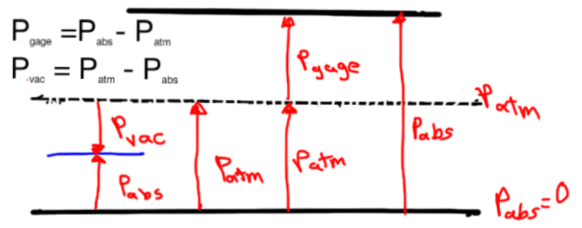
Pressure Measurement
![]()
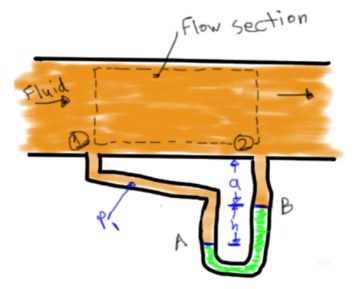
Pressure Relation From Point A-B
![]()
Pressure Difference
![]()
![]()
![]()
| Formula | Units | |
|---|---|---|
| Newton’s Second Law |
|
|
| Weight |
|
|
| Density |
|
|
| Specific Volume |
|
|
| Specific Weight |
|
|
| Temperature Conversion |
|
![]()
![]()
![]()