What if we have a fractional compound or discount rate – such as 9.5% – which is not to be found in published tables? Can we still use the tables? Or do we need to solve the problem mathematically (or by financial calculator)?   

One way to get around this is by estimating the multiplier by means of averaging the two whole multipliers that bracket the fractional one in question. We call this process “interpolation” because we insert the average number in between the relevant values or factors, which are stated in the table. Let’s use the future value table below to illustrate this.

Future Value Factors

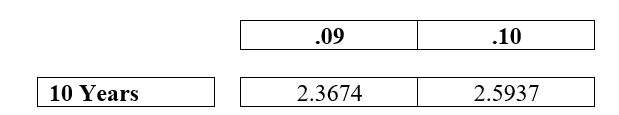
What is the appropriate, estimated future value multiplier for 10 years at 9.5%? This can be approximated by taking the simple average of the multipliers for 9% and 10%: 

(2.3674 + 2.5937) ÷ 2 = 2.4806 

This is only an estimate. Remember that time value factors increase (in this case – or decrease in the case of present values) at an exponential rate. The true mathematical future value for 9.5% for 10 years is: 

1.095¹⁰ = 2.4782 

This differs from the simple average estimate, of 2.4806 – 2.4782 = 0.002. We first note that the simple average produces a higher number than the true, exponential figure; this is notable. This is as expected because, in dealing with future value factors, the numbers grow exponentially or more quickly, and thus we would start off with a lower number. Put differently, we do not grow from 9% to 10% at a linear rate, but at an increasing, curvilinear rate.